
DonCorson
[AHCI]
3358

Resonance Watches, a First Approach
Resonance Watches, a First Approach
by Don Corson
Resonance watches are rare and when discussed the resonance effect is often not accepted. Always looking for a challnge, I have decided to make my next watch a resonance watch to see what is real and what is only talk. While the mechanical design of the movement has slowly been advancing I have also been interested in the theoretical side of the phenomenon of resonance. In other domains we use resonance daily without even knowing it probably, why shouldn’t it work in mechanical watches? (Note that I realise that resonance is the wrong term technically. We should be talking about synchronisation of coupled oscillators. I will, however, retain the term resonance for historical reasons. Antide Janvier and A.L. Breguet called their clocks and watches with coupled oscillators resonant timekeepers. Journe and Haldimann do too. So will I.)
The only modern resonance watches that I know of are the F.-P. Journe Chronomètre à Résonance and the Haldimann H2 Flying Resonance. There are several other recent multi-oscillator watches such as the new MB&F Legacy 2 and the Dufour Duality, but these watches do not try and make use of the resonance effect, they simply average the rate of the two or more oscillators.


F.-P. Journe Chronomètre à Résonance
While planning this resonance watch I have looked into the tools that are available to see what kind of performance can be expected. As all of the analytical treatments of the watch oscillator and escapement that I know treat the subject in a steady state, certainly true, but missing much of real life, I decided to skip the analytical treatment and will try to make a “similar to the real world” simulation. The advancement of simulation tools in the last 10 years gives us this possibility with easy to use and reliable simulation environments.
The first step is to make a simple simulation of the oscillator of a wrist watch, the balance wheel / hairspring pair. In this oscillator the energy is transferred between 2 storage units. If it would be possible to eliminate all losses the oscillator once started would keep transferring the energy between the kinetic energy of the balance and its speed and the potential energy of the hairspring and its position forever. The kinetic energy of the balance at its fastest arc speed would be equal to the potential energy of the hairspring at its greatest extension or compression.
In the real world we must take into account at least the linear friction that is proportional to the arc speed of the balance wheel. For my first attempt I will ignore the viscous friction that is proportional to the acceleration of the balance.
Below is a typical model of a simple oscillating system.

The friction of the balance axle, the spring constant of the hairspring and the inertia of the balance wheel are the parameters that need to be determined and confirmed in reality.
Starting this model with an initial displacement of 120 degrees shows us a result as follows:
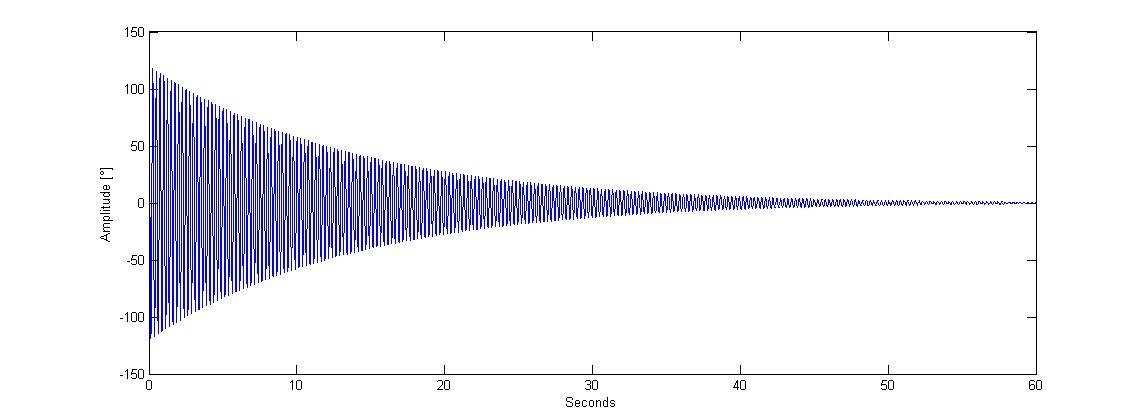
(The vertical axis is the amplitude in degrees, the horizontal axis time in seconds.)
We see a decreasing amplitude that I can now compare with a real unit to correct the parameter for friction. This comparison with an ETA 2824-A2 movement I had laying around showed that I need to increase the friction as the oscillations of the real balance wheel decrease somewhat faster than that in figure 2.
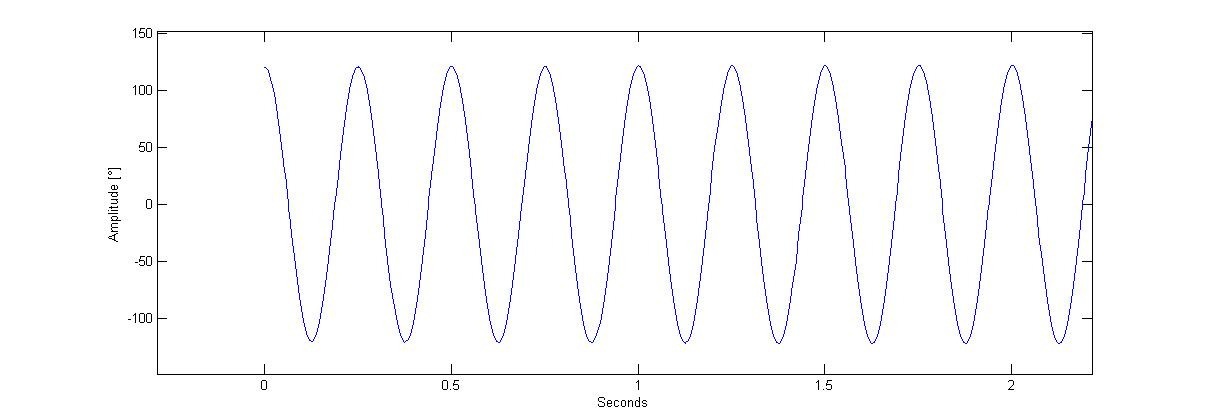
In a zoomed section we can see that the frequency is correct. There are 4 complete oscillations in each second. As such the balance inertia and spring constant appear to be correct.
The next thing that needs to be added to the above model is the excitation of the balance wheel, a simulation of the impulse from the anchor. As a first approach I have added a force to those acting on the balance wheel. That force acts during the 50 degrees centered around the position 0, just as it is in a real watch. With the addition of the impulse the figure 2 becomes the figure 4 below:
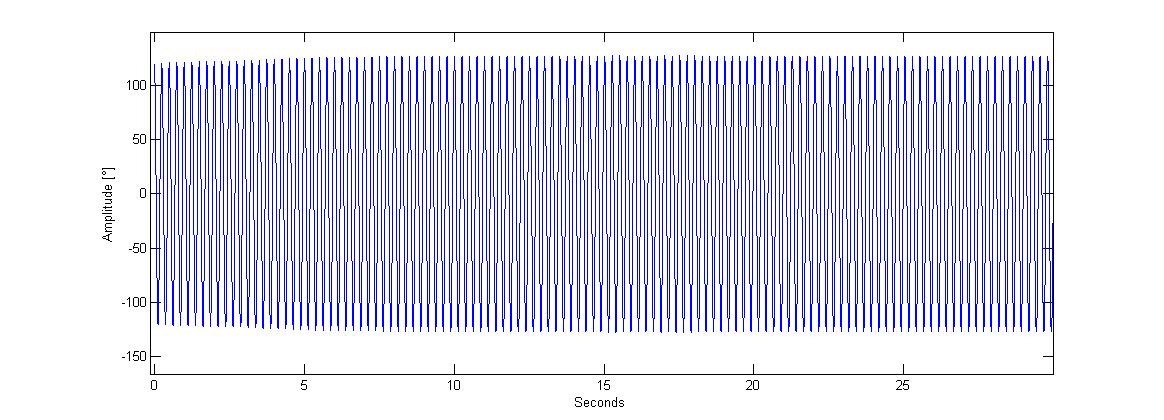
The energy added by the impulse keeps the amplitude from falling off. The amplitude stabilises at about 280 degrees.
This model has a problem for with I have not seen a solution in the literature. The anchor is not only a means for adding energy to the balance wheel through the impulse, but if external forces act on the balance so it has a high amplitude or speed the anchor also serves to slow the balance down, to stabilise the amplitude in a normal region. For the moment I have added a feature to the model so that if the speed of the balance is faster than for a 300° amplitude the impulse is not given. This does not correspond well with reality which actually slows the balance, but is a first step in that direction. I will think about this problem some more to make a more realistic simulation in the future.
Now that I have an oscillating system that corresponds more or less to a real balance wheel/ hairspring oscillator it is time to see how it is affected by oscillations coming from an external source. How will this model act as a part of a resonant system? I will make this first simulation with a simple sine wave generator and couple the energy from this source into the balance wheel. There will be no feedback from the balance wheel to the sinus generator as there would be in a resonance watch. But we want to take small steps and try to understand things on the way.
What do I expect? Well, I expect that at frequencies far from the natural oscillating frequency of the balance the external excitation will have little influence on the balance wheel. At frequencies near or at the balances natural oscillating frequency there will be a big increase in the amplitude of the balance as the balance resonates with the external excitation.
To do this simulation have reduced the impulse so the amplitude does not become excessive. I will run the model with several external excitation frequencies with an energy somewhat less to that used for the excitation from the anchor and note the amplitude of the balance wheel when it has arrived at a stable state.
Here are the results. The horizontal axis shows the frequency of the external excitation, the vertical axis the amplitude after the system stabilised. The model needs about 60 seconds to stabilise. It will be interesting to see if the real watch has the same behaviour. The values were taken after 120 seconds.

With this we have seen that a simulation can give us some insight into the behaviour of a resonant watch. I have two major questions now:
- How is the frequency behaviour in a resonant state?
- How does the system react to disturbances, one time shocks, for example?
I will next investigate this and compare the behaviour with a simple oscillator.
Then I will make the system of two balance wheels with a coupling spring between them and more questions will come up.
- What is the ideal coupling factor between the oscillators?
- Is the system more stable when the oscillators are in phase, both balances turning in the same direction, or out of phase, the balances turning in opposite directions, or does it make no difference?
- …..
At the same time I am starting to make the production drawings for the watch and hope to start making it “in the metal” soon to be able to see if the simulation has any relationship with reality. Preparing the means for testing the real movement will also take some time. A standard timing machine will not work as it uses the sounds made by the escapement to do the timing. A watch with two different escapements working at the same time will confuse the timing machine completely. I will have to make an optical timing system to be able to follow the two oscillators independently.
Lots to do.

This message has been edited by DonCorson on 2013-10-29 05:36:27


